How do you find the center of rotation with tracing paper?

Tracing paper is a useful tool in determining the center of rotation in various contexts. Whether you are working with a geometric shape, a physical object, or a mathematical problem, tracing paper can help you identify the precise point around which an object rotates.

To find the center of rotation with tracing paper, you will need a few materials: a piece of tracing paper, a pencil, and the object or shape you want to examine. Begin by placing the tracing paper over the object.

Using your pencil, trace the outline of the object onto the paper. Ensure that you make clear and accurate marks to obtain an exact replica of the shape.

Next, choose a point on the traced shape that you believe might be the center of rotation. Place the tip of your pencil on this point and make an arrow or line segment extending outwards from the point in any direction.

Now, carefully rotate the tracing paper around the object, but keep the pencil firmly on the arrow or line segment you just drew. As you rotate, observe whether the rest of the traced shape aligns with the original object. If it does, congratulations! You have found the correct center of rotation.

If the traced shape doesn't align with the object, it means that you haven't placed the center of rotation correctly. In this case, adjust the point you chose earlier and repeat the process until the alignment is perfect.

Remember to take your time and be patient while using tracing paper to find the center of rotation. It may take a few attempts, particularly if the shape is complex or irregular. However, with practice and attention to detail, you will become proficient in using this technique.

In conclusion, tracing paper provides a visual and hands-on approach to identifying the center of rotation. By tracing the shape and carefully observing the alignment while rotating the paper, you can confidently determine the precise point around which an object rotates.

How do you find the center of rotation on paper?

When working with paper, finding the center of rotation is essential for tasks such as creating symmetrical designs or measuring rotations accurately. There are a few techniques you can use to determine the center of rotation on paper.

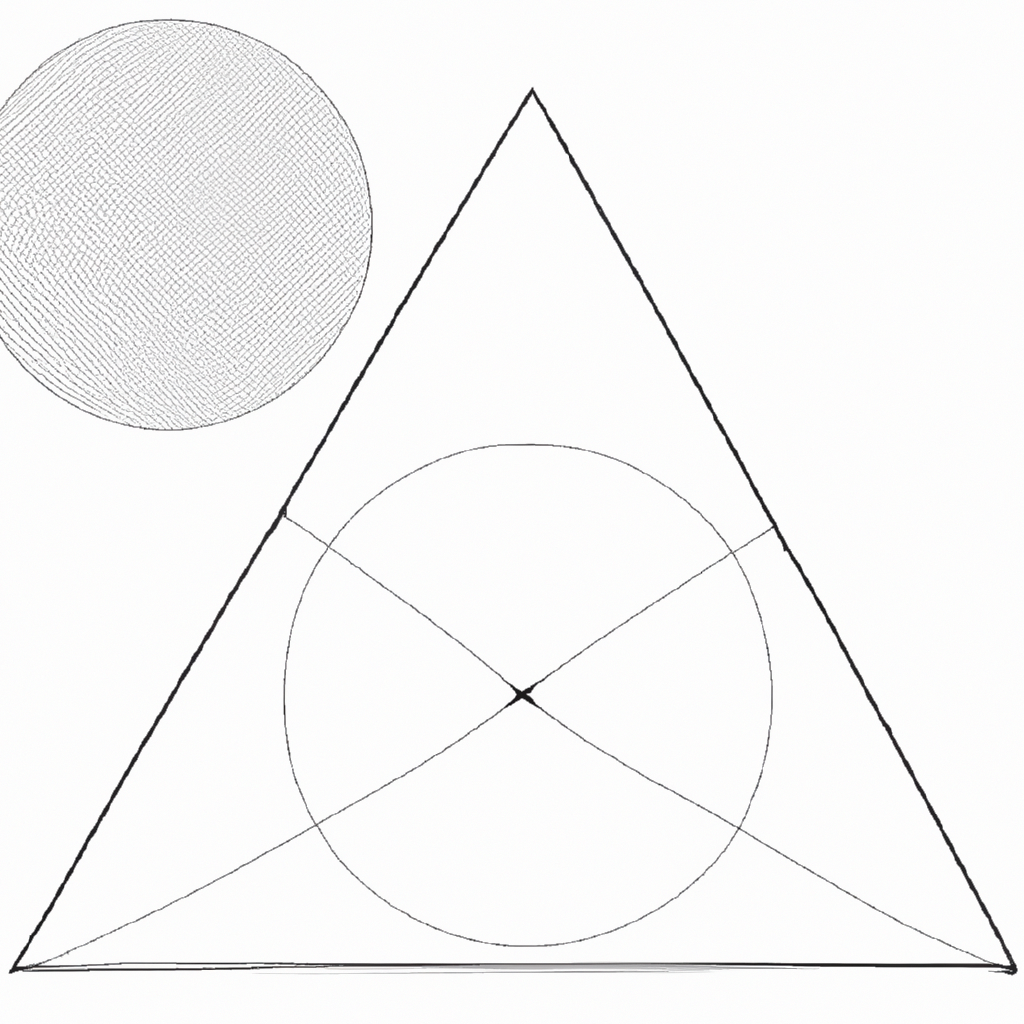
One method is by using a compass. Start by drawing a circle on the paper. Then, choose a point on the circle and use the compass to draw arcs intersecting the circle at two different points. Repeat this process with another point on the circle. The center of rotation will be where these arcs intersect. You can mark it with a dot or a small cross.

To verify the accuracy of the center of rotation, you can rotate the paper around the marked point. If the paper rotates smoothly and evenly, you have found the correct center of rotation.

Another technique to find the center of rotation is by using geometrical shapes. Draw two lines on the paper, intersecting each other at a point. Then, draw a third line that is perpendicular to the intersection point. Measure and mark equal distances on all three lines. The center of rotation will be where these marked distances align. You can indicate it with a small circle or dot.

If you want to be more precise, you can measure and mark several equal distances on each line and find the point where these segments intersect. This will provide a more accurate center of rotation.

A different approach to finding the center of rotation is by using known reference points. If you have objects or shapes on your paper that already have a defined center, you can use them as reference points. Measure and mark equal distances from these reference points and look for the intersection. This will help you locate the center of rotation more easily.

It's important to remember that finding the center of rotation requires precision and careful measurement. Take your time and double-check your calculations if needed. Once you have determined the center of rotation, you can utilize it for various purposes in your paper-based creations.

How do you find the center point of a rotation?

When it comes to finding the center point of a rotation, there are a few key steps to follow. *First, you need to determine the axis of rotation. This is the line or point around which the object will rotate. This can be a vertical or horizontal line, or even a specific point in three-dimensional space.

Once you have identified the axis of rotation, the next step is to find a reference point on the object that will serve as the center of rotation. *Typically, this reference point is labeled as the "origin" or "center" of the object. It is a fixed point that will remain stationary during the rotation.

In order to find this reference point, you can use *different methods depending on the object and the situation. For example, if you have a geometric shape like a square or a circle, the reference point will be the exact center of the shape. This can be easily determined by measuring the width and height of the object and calculating the midpoint.

*In more complex situations, where the object is irregular in shape or has uneven weight distribution, it may be necessary to use additional tools or techniques to locate the reference point. This might involve using a balance to determine the center of mass or performing physical experiments to identify the point around which the object rotates most easily.

Once you have determined the reference point or center of rotation, you can then apply the necessary *mathematical calculations or computer algorithms to perform the rotation accurately. These calculations will typically involve the coordinates of the reference point, as well as the angle and direction of rotation.

In conclusion, finding the center point of a rotation requires identifying the axis of rotation and locating the reference point or center of rotation on the object. With the appropriate measurements and calculations, you can then perform the rotation accurately and achieve the desired outcome.

How do you use tracing paper for rotational symmetry?

Rotational symmetry is a concept in mathematics and design where an object remains unchanged or looks the same after a certain rotation. It is an important element used in many fields such as art, architecture, and engineering. One tool that can be used to explore and create rotational symmetry is tracing paper.

Tracing paper is a translucent paper that allows you to see through it. It is commonly used for tracing and copying images. When it comes to exploring and creating rotational symmetry, tracing paper can be a helpful tool. Here's how you can use it:

1. Start by selecting or creating a shape that you want to explore or create rotational symmetry with. It can be anything from a simple geometric shape to a more complex design. Draw the shape on a piece of regular paper or print it out if you have a digital version.

2. Place the tracing paper on top of the shape you have drawn or printed. Make sure the tracing paper covers the entire shape and is secured in place. You can use tape or paper clips to hold it in place if needed.

3. Rotate the tracing paper around a certain point or axis. You can start by rotating it in small increments, such as 30 degrees at a time, to see how the shape changes with each rotation. You can also experiment with rotating it at different angles or multiples of the same angle.

4. Observe the results as you rotate the tracing paper. Notice how the shape appears to change or repeat itself after each rotation. You may also discover new patterns or symmetrical arrangements that emerge as you rotate the paper.

5. Experiment with different shapes and rotations to explore the possibilities of rotational symmetry further. Try combining multiple shapes or rotating the tracing paper around different points to create more complex symmetrical designs.

Using tracing paper for exploring and creating rotational symmetry allows you to visually understand and manipulate patterns and designs. It is a hands-on approach that can be both educational and enjoyable. So, grab a piece of tracing paper and start unlocking the world of rotational symmetry!

How do you rotate an object using tracing paper?

Rotating an object using tracing paper is a simple and effective technique that can help you accurately measure and replicate angles. This method is commonly used in various fields such as engineering, architecture, and art.

The first step in rotating an object using tracing paper is to place the tracing paper over the object you wish to rotate. Make sure the paper is aligned properly and secured in place to prevent any movement.

Now, select a point on the object around which you want to rotate it. This point is commonly referred to as the center of rotation and serves as the anchor point for the rotation. Choose a spot that is easily identifiable on both the object and the tracing paper.

Next, use a pencil or pen to mark the center of rotation on both the object and the tracing paper. This will ensure that you have a clear reference point for the rotation.

Once you have marked the center of rotation, select an angle by which you want to rotate the object. This can be any desired angle, whether it's 90 degrees, 45 degrees, or any other specific measurement. Using a protractor or angle ruler, carefully measure and mark the desired angle on the tracing paper.

Now, you can begin the rotation. Keeping the center of rotation as the anchor point, gently rotate the object in the desired direction until the angle marked on the tracing paper aligns with the object.

Throughout the rotation process, ensure that the tracing paper remains securely in place. This will help maintain the accuracy of the rotation and prevent any unwanted movements or misalignments.

Once you have completed the rotation, you can remove the tracing paper. The object will now be rotated to the desired angle, and you can use this as a reference for further measurements, drawings, or any other tasks.

In conclusion, rotating an object using tracing paper is a practical technique that allows for precision and accuracy. By following the steps outlined above, you can easily rotate an object to any desired angle and create accurate measurements and drawings.